Reconsidere o . Usando EES (ou outro programa), investigue o efeito da temperatura da base da aleta sobre a temperatura na ponta e sobre a taxa de transferência de calor a partir da aleta. Deixe a temperatura na base da aleta variar de a . Trace a temperatura da ponta da aleta e a taxa de transferência de calor em função da temperatura da base e discuta os resultados.
Passo 1
Realizando o algoritmo em duas partes, uma sendo dados e outra analise temos que:
"DADOS"
k=180 [W/m-C]
L=0.05 [m]
b=0.01 [m]
w=1 [m]
T_0=180 [C]
T_amb=25 [C]
h=25 [W/m^2-C]
T_cir=290 [K]
M=6
epsilon=0.9
tan(theta)=(0.5*b)/L
sigma=5.67E-8 [W/m^2-K^4] “constante de Stefan-Boltzmann"
"ANÁLISE"
"letra (a)"
DELTAx=L/(M-1)
"equacionamento a partir do método das diferenças finitas"
“nó 1”
(1-0.5*DELTAx/L)*(T_0-T_1)+(1-1.5*DELTAx/L)*(T_2-T_1)+(h*DELTAx^2)/(k*L*sin(theta))*(T_amb-T_1)+(epsilon*sigma*DELTAX^2)/(k*L*sin(theta))*(T_cir^4-(T_1+273)^4)=0
“nó 2”
(1-1.5*DELTAx/L)*(T_1-T_2)+(1-2.5*DELTAx/L)*(T_3-T_2)+(h*DELTAx^2)/(k*L*sin(theta))*(T_amb-T_2)+(epsilon*sigma*DELTAX^2)/(k*L*sin(theta))*(T_cir^4-(T_2+273)^4)=0
“nó 3”
(1-2.5*DELTAx/L)*(T_2-T_3)+(1-3.5*DELTAx/L)*(T_4-T_3)+(h*DELTAx^2)/(k*L*sin(theta))*(T_amb-T_3)+(epsilon*sigma*DELTAX^2)/(k*L*sin(theta))*(T_cir^4-(T_3+273)^4)=0
“nó 4”
(1-3.5*DELTAx/L)*(T_3-T_4)+(1-4.5*DELTAx/L)*(T_5-T_4)+(h*DELTAx^2)/(k*L*sin(theta))*(T_amb-T_4)+(epsilon*sigma*DELTAX^2)/(k*L*sin(theta))*(T_cir^4-(T_4+273)^4)=0
“nó 5”
2*k*DELTAx/2*tan(theta)*(T_4-T_5)/DELTAx+2*h*(0.5*DELTAx)/cos(theta)*(T_amb-T_5)+2*epsilon*sigma*(0.5*DELTAx)/cos(theta)*(T_cir^4-(T_5+273)^4)=0
T_ponta=T_5
"letra (b)"
Q_ponto_aleta=C+D "onde"
C=h*(w*DELTAx)/cos(theta)*((T_0-T_amb)+2*(T_1-T_amb)+2*(T_2-T_amb)+2*(T_3-T_amb)+2*(T_4-
T_amb)+(T_5-T_amb))
D=epsilon*sigma*(w*DELTAx)/cos(theta)*(((T_0+273)^4-T_cir^4)+2*((T_1+273)^4-T_cir^4)+2*((T_2+273)^4-
T_cir^4)+2*((T_3+273)^4-T_cir^4)+2*((T_4+273)^4-T_cir^4)+((T_5+273)^4-T_cir^4))
Passo 2
Realizando a tabela e preenchendo com as interações:
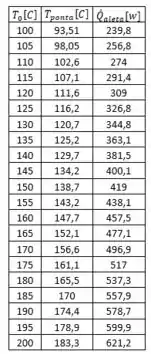
Logo abaixo a representação gráfica:
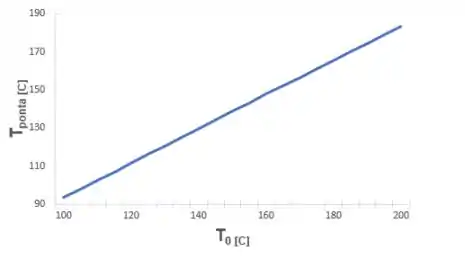
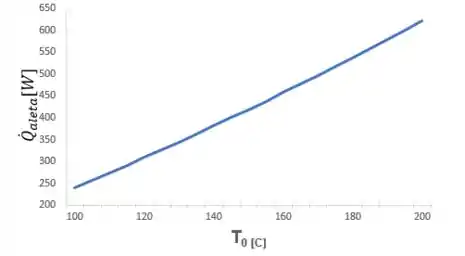
Resposta
Ei, a resposta está no passo a passo :)