Responde Aí
Acesse com GoogleAcesse com AppleAcesse com Facebook

Enunciado

FenTrans, MecFlu, TransCal e TransMassa - Çengel - Mecânica dos Fluidos- Ed: 1º - Capítulo 12.Problemas - Ex. 130

Vapor a 6,0 MPa e 700 K entra em um bocal convergente com velocidade desprezível. A área da garganta do bocal é de 8 cm². Assumindo um fluxo isentrópico, faça um gráfico da pressão de saída, a velocidade de saída e a vazão em massa através do bocal versus a contrapressão P b para 6,0 ≥ P b ≥ 3,0   M P a . Trate o vapor como um gás ideal com k = 1.3 ,   c p = 1,872 k J k g K   e   R = 0,462 k J k g K .

Passo 1

Vamos assumir que o fluxo neste caso é constante, unidimensional e isentrópico e o ar se comportará como gás ideal !!

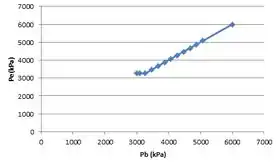
Aqui é muito importante nós acharmos a pressão crítica!! Porque para todas as contrapressões inferiores a P * , a pressão no plano de saída do bocal é igual a ela, ou seja:

P e = P *             P b < P *

P e = P b             P b ≥ P *

Então aqui nós já achamos uma forma de calcular a variação da pressão na saída !!

Lembrando que:

P * = P 0 2 k + 1 k k - 1

A temperatura e a pressão de estagnação, assumindo a velocidade desprezível, são dadas por:

T 0 = T 1 + V 1 2 2 c p             ∴           T 0 = T 1

P 0 = P 1 T 0 T 1 k k - 1             ∴           P 0 = P 1

Para o vapor nós temos que:

k = 1.3

c p = 1872 J k g K

R = 462 J k g K

E que:

V 1 = 0 m s

P 1 = 60 00   k P a

T 1 = 7 00   K

Então:

T 0 = 7 00   K

P 0 = 6000   k P a

E a pressão de estagnação:

P * = 6000   k P a 2 1 , 3 + 1 1 , 3 1 , 3 - 1 = 3274,4   k P a

Então:

P e = 3274,4             P b < 3274,4   k P a

P e = P b             P b ≥ 3274,4 k P a

Fazendo um gráfico com essas informações:

Passo 2

A temperatura é dada por:

T e = T 0 P e P 0 k - 1 k

Então:

T e = 700   K 3274,4   k P a 6000   k P a 1 , 3 - 1 1 , 3               P b < 3274,4   k P a

T e = 700   K P b 6000   k P a 1,3 - 1 1,3               P b ≥ 3274,4   k P a

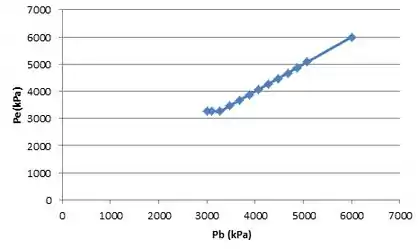
Como queremos a velocidade basta nós lembramos da fórmula:

V = 2 c ´ p T 0 - T e

Igual fizemos para a anterior:

V = 2 1872 J k g . K 700   K - 608,7   K               P b < 3274,4   k P a

V = 2 1872 J k g . K 700   K - T e               P b ≥ 3274,4   k P a

E no gráfico:

Passo 3

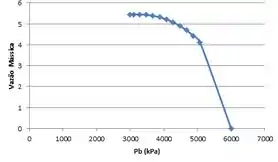
E para finalizar, a vazão mássica:

m ˙ = ρ e V e A e = P e R T e V e A e

Então:

m ˙ = 3274,4 × 10 3 P a 462 J k g . K 608,7   K 584,7 m s 0,00 08   m 2         P b < 3274,4   k P a

m ˙ = P e 462 J k g . K T e V e 0,00 08   m 2         P b ≥ 3274,4 k P a

E assim:

Aguardo vocês na próximaa !!!

Resposta

Ver Outros Exercícios desse livro

Exercícios de Livros Relacionados

Water flows in a brickwork rectangular channel 2 m wide,on a slope of 5 m/km. (a) Find the fl ow rate when thenormal depth is 50 cm. (b) If the normal depth remains50 cm, fi nd the channel width which
An unfinished concrete sewer pipe, of diameter 4 ft, isflowing half-full at 39,500 U.S. gallons per minute. If thisis the normal depth, what is the pipe slope, in degrees?
It is desired to excavate a clean-earth channel as a trapezoidal cross section with θ 5 608 (see Fig. 10.7). The expectedflow rate is 500 ft3/s, and the slope is 8 ft per mile. Theuniform flow depth i
For more than a century, woodsmen harvested trees inSkowhegan, ME, elevation 171 ft, and fl oated the logsdown the Kennebec River to Bath, ME, elevation 62 ft, adistance of 72 miles. The river has an
Determine the most effi cient dimensions for a clay tilerectangular channel to carry 110,000 gal/min on a slopeof 0.002.