Enunciado
FenTrans, MecFlu, TransCal e TransMassa - Frank White - Mecânica dos Fluidos- Ed: 6º - Capítulo 1.Problemas - Ex. 83
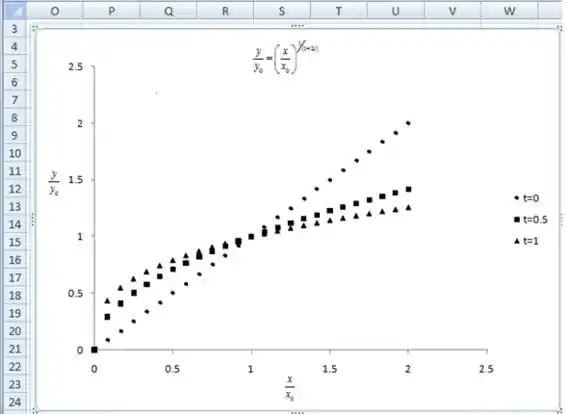
Um campo de velocidade não permanente bidimensional é dado por , . Encontre a equação das linhas de corrente variáveis no tempo, que passam, todas, através do ponto em algum instante . Esboce algumas delas.
Passo 1
Bem, vamos lembrar que a equação apresentada é uma equação diferencial de segunda ordem, então podemos organizar ela para ela tomar essa cara!
Passo 2
Agora basta integrarmos nossa equação:
Onde é uma constante!
Pra matar de vez, temos que:
Aplicando a função inversa, temos!
Para garantir, que todas as linhas passem por devemos agrega-los na equação como parâmetros, assim:
Para esboço do gráfico recomendo o uso do webapp do geogebra, ou o do wolfran para plot de gráficos em 3d!
Isso é tudo pessoal, conseguimos mais uma! \o/
Passo 3
Resposta
Exercícios de Livros Relacionados
In the early 1900s, the British chemist Sir CyrilHinshelwood quipped that fl uid dynamics study was divided into “workers who observed things they could notexplain and workers who explained things the
Data for the apparent viscosity of average human blood, atnormal body temperature of 378C, varies with shear strainrate, as shown in the following table.Strain rate, s21 1 10 100 1000Apparent viscosit
From Table A.3, the density of glycerin at standard conditionsis about 1260 kg/m3. At a very high pressure of 8000 lb/in2, itsdensity increases to approximately 1275 kg/m3. Use this datato estimate th
A right circular cylinder volume υ is to be calculated fromthe measured base radius R and height H. If the uncertaintyin R is 2 percent and the uncertainty in H is 3 percent, estimate the overall unce
Assume that the n-pentane data of Prob. P1.36 representsisentropic conditions. Estimate the value of the speed ofsound at a pressure of 30 MPa. [Hint: The data approximately fi t Eq. (1.19) with B 5 2