Responde Aí
Acesse com GoogleAcesse com AppleAcesse com Facebook

Enunciado

FenTrans, MecFlu, TransCal e TransMassa - Fox - Mecânica dos Fluidos- Ed: 8º - Capítulo 11.Problemas - Ex. 51

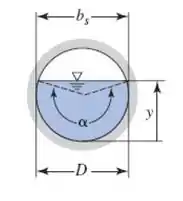
Uma cuba semicircular de aço corrugado, com diâmetro D = 1   m , carrega água a uma profundidade y = 0,25   m . A inclinação é de 0,01. Determine a descarga.

Passo 1

Beleza! Para podermos encontrar a descarga, dada pela equação:

Q = 1 n A R h 2 3 S b 1 2

Precisamos encontrar todos esses parâmetros antes. Então, vamos começar com a geometria do canal, sabemos, do esquema do enunciado, que α será:

α = 2 sin - 1 ⁡ y - D 2 D 2 + 180 °

Substituindo os dados conhecidos, temos:

α = 2 sin - 1 ⁡ 0,25 - 1 2 1 2 + 180 ° → α = 120 °

Passo 2

Agora, com os valores dados no enunciado e o valor de α , com o auxílio da tabela de geometria a seguir, conseguimos encontrar os termos que precisamos:

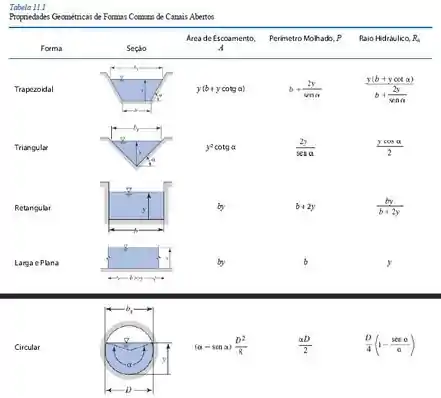
Então, para o nosso caso, teremos (lembrando de utilizar o ângulo em radianos):

A = α - s e n α D 2 8 = 2,09 - s e n 2,09 1 2 8 → A = 0,153   m 2

R h = D 4 1 - s e n α α = 1 4 1 - s e n 2,09 2,09 → R h = 0,146   m

Então, agora só falta obter o valor de n , para isso, vamos recorrer a tabela a seguir:

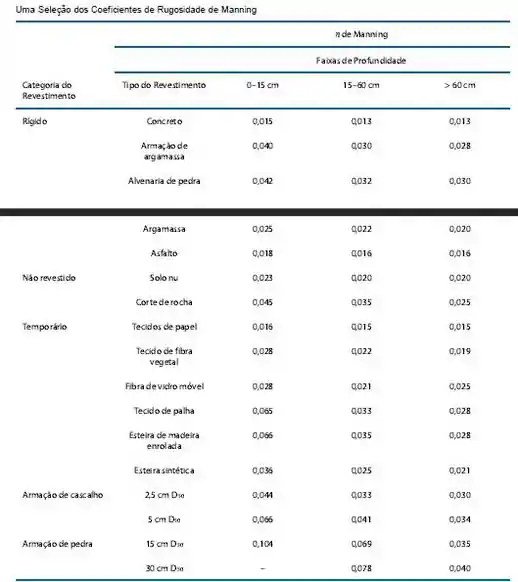
Beleza, não temos o valor do n para o aço corrugado, então, pesquisando na internet, obtemos um valor de:

n = 0,02

Passo 3

Então, a equação para a descarga será:

Q = 1 n A R h 2 3 S b 1 2 = 1 0,02 0,153 0,146 2 3 0,01 1 2 → Q = 0,212   m 3 / s

Resposta

Q = 0,212   m 3 / s

Ver Outros Exercícios desse livro

Exercícios de Livros Relacionados

For a half-hexagon channel running full, fi nd an analyticexpression for the propagation speed of a small-disturbancewave travelling along this channel. Denote the bottomwidth as b and use Fig. 10.7 a
In Fig. P10.62, let Vo 5 5.5 m/s and yo 5 90 cm. (a) Will thewater rise or fall over the bump? (b) For a bump height of30 cm, determine the Froude number over the bump. (c) Findthe bump height that wi
For the rectangular channel in Prob. P10.60, the Froudenumber over the bump is about 1.37, which is 17 percentless than the approach value. For the same entrance conditions, fi nd the bump height Dh t
A 4-ft-diameter fi nished-concrete sewer pipe is half full ofwater. (a) In the spirit of Fig. 10.4a, estimate the speed ofpropagation of a small-amplitude wave propagating alongthe channel. (b) If the
Modify Prob. P10.63 so that the 15-cm change in bottomlevel is a depression, not a bump. Estimate (a) the Froudenumber above the depression and (b) the maximum changein water depth.