Enunciado
Cálculo - Guidorizzi - Vol 3- Ed: 5º - Capítulo 4.2 - Ex. 1j
Calcule
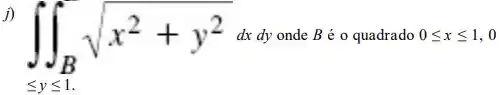
Passo 1
Observando o conjunto deseja-se calcular a integral dupla através da mudança de variável. Lembrando que, pelo teorema de Fubini:
Ou:
Neste caso, é dado no enunciado como um quadrado e pode ser resolvido através de coordenadas polares na mudança de variável. Então, fazendo:
Pretende-se determinar o resultado da transformação:
Dada por:
Então:
Portanto:
Passo 2
Agora, deve se determinar o conjunto , onde . Assim, o novo conjunto será dado através do limite do conjunto original. A partir de , as novas variáveis serão dadas por:
Quando
Quando
Quando
Quando
Ou seja, haverão duas integrais sendo cada uma para cada intervalo de .
E, para o intervalo relacionado à variável :
Já para o intervalo relacionado à variável :
Dessa forma, tem-se:
Passo 3
Logo, mudando a variável na integral dupla:
E, resolvendo-a:
Substituindo o intervalo de integração:
Passo 4
Para ambos os termos, utiliza-se o método da integral por partes, tal que para o primeiro:
e e
E para o segundo:
e e
Lembrando que o método é dado por:
Então:
Assim:
Similarmente, o segundo termo:
Assim:
Então, substituindo na integral inicial:
Substituindo o intervalo de integração:
Como :