Responde Aí
Acesse com GoogleAcesse com AppleAcesse com Facebook

Enunciado

Mecânica e Resistência dos Materiais - Mecânica para Engenharia Estática - 12ª Edição - Russel C. Hibbeler- Ed: 12º - Capítulo 9.Problemas - Ex. 29

Localize o centroide overset(-)(y) da área.

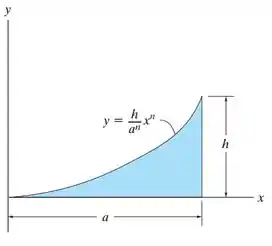
Passo 1

Vamos tomar nosso elemento diferencial como a área em cinza mostrada na figura:

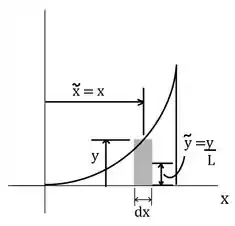
Essa área é dada por:

d A = y ⅆ x

Tendo nossa função y = (h)/(a^n) x^n , nosso d A fica:

d A = (h)/(a^n) x^n d x

Para o centroide do nosso elemento diferencial, em overset(-)(y) :

overset(-)(y) = (y)/(2)

overset(-)(y) = (1)/(2) (h)/(a^n) x^n

Passo 2

Vamos encontrar nossa área:

A = int_A ⅆ A

A = int_0^a ((h)/(a^n) x^n) ⅆ x = [(h x^(n + 1))/(a^n ( n + 1 ))]_0^a = (h a^(n + 1))/(a^n ( n + 1 ))

Passo 3

E agora encontramos nosso centroide overset(-)(y) :

overset(-)(y) = (int_A overset(-)(y) ⅆ A)/(int_A ⅆ A)

Onde:

int_A overset(-)(y) ⅆ A = int_0^a ((1)/(2) (h)/(a^n) x^n) ((h)/(a^n) x^n) ⅆ x = (1)/(2) int_0^a ((h^2)/(a^(2 n)) x^(2 n)) ⅆ x

= (1)/(2) [(h^2 x^(2 n + 1))/(a^(2 n) ( 2 n + 1 ))]_0^a = (h^2 a)/(2 ( 2 n + 1 ))

Logo, nosso overset(-)(y) fica:

overset(-)(y) = ((h^2 a)/((2 n + 1)))/((h a^(n + 1))/(a^n (n + 1))) :. overset(-)(y) = (n + 1)/(2 ( 2 n + 1 )) h

Resposta

overset(-)(y) = (n + 1)/(2 ( 2 n + 1 )) h

Ver Outros Exercícios desse livro

Exercícios de Livros Relacionados

Determine o centróide da em z da figura abaixo.
With your pencil, make a dot on the position of your best visual estimate of the centroid of the area of the circular sector. Check your estimate by using the results of Sample Problem 5/3.
Specify the x-, y-, and z-coordinates of the mass center of the homogeneous semicylinder.
With your pencil, make a dot on the position of your best visual estimate of the centroid of the triangular area. Check the position of your estimate by referring to the results of Sample Problem 5/2
Determine the y-coordinate of the centroid of the shaded area. Check your result for the special case a = 0.