Responde Aí
Acesse com GoogleAcesse com AppleAcesse com Facebook

Enunciado

Cálculo - Cálculo Volume 1 - 5ª Edição - James Stewart- Ed: 5º - Capítulo 7.3 - Ex. 21

Avalie a integral.

int_0^((2)/(3)) x^3 sqrt(4 - 9 x^2) d x

Passo 1

Para a resolução de uma integral pela substituição trigonométrica, precisamos fazer a escolha da substituição. Sendo 4 x = 3 sec theta , onde 0 <= theta <= (pi)/(2) . Logo, derivando temos d x = (3)/(4) sec theta d theta .

Para sqrt(16 x^2 - 9) , temos que

sqrt(16 ( (3)/(4) sec theta )^2 - 9) = sqrt(9 sec^2 theta - 9) = 3 sqrt(sec^2 theta - 1) = 3 tan theta

Assim podemos substituir!!

int ((3)/(4) sec theta d theta)/(( (3 sec theta)/(4) )^2 3 tan theta)

Simplificamos a integral e teremos:

(4)/(9) int cos theta d theta

Essa integral tem como resultado:

(4)/(9) int cos theta d theta = (4)/(9) sin theta + C

Passo 2

Esboçando o triangulo retângulo associado, temos:

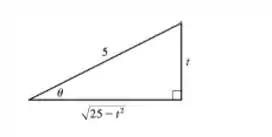
Então,

int (d x)/(x^2 sqrt(16 x^2 - 9)) = (4)/(9) (sqrt(16 x^2 - 9))/(4 x) + C = (sqrt(16 x^2 - 9))/(9 x) + C

Prontinho!!

Resposta

int (d x)/(x^2 sqrt(16 x^2 - 9)) = (4)/(9) (sqrt(16 x^2 - 9))/(4 x) + C = (sqrt(16 x^2 - 9))/(9 x) + C

Ver Outros Exercícios desse livro

Exercícios de Livros Relacionados

c)int c o s (2 x) cos (x) d x
e) ∫ - π π s e n n x cos ⁡ m x d x
a) ∫ s e n 7 x cos ⁡ 2 x d x
b)int s e n (3 x) sen (5 x) d x
3) Calcule int_(- pi)^(pi) s e n (n x) s e n (m x) d x , sendo m e n naturais não-nulos.