Calculeint (1)/(x sqrt(1 + x^2)) d x

int (1)/(x sqrt(1 + x^2)) d x = ln |(sqrt(1 + x^2) - 1)/(x)| + k

Enunciado

A função dentro da integral é:

Não parece muito familiar?

Na verdade a resolução pra esse tipo de função é chamada substituição trigonométrica e funciona assim:

A gente pega um triângulo retângulo com ângulo como esse:

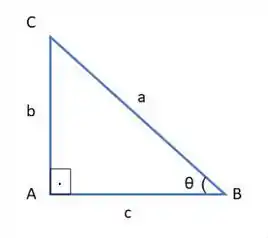
Agora imagine que um dos catetos vale 1, e o outro cateto vale , concorda comigo que a hipotenusa vai valer ?

Então que substituição poderíamos fazer?

Bem, se dissermos que então

Assim podemos substituir a integral dessa forma:

Ótimo! Agora podemos calcular essa integral de maneira mais fácil

Agora só precisamos achar o valor de

Pra isso vamos fazer assim:

Que é exatamente:

Podemos substituir pela variável

Assim:

Substituindo, teremos:

Agora precisamos voltar pra variável , lembrando:

E que:

Assim: