Enunciado
Cálculo - Cálculo Volume 1 - 6ª Edição - James Stewart- Ed: 6º - Capítulo 7.3 - Ex. 7
Calcule a integral.
Passo 1
O triângulo retângulo associado a esse desenho é o seguinte:
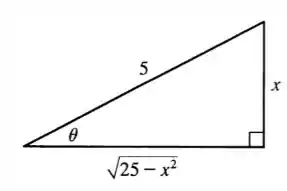
Passo 2
A gente tem que , e sua derivada é .
Passo 3
Assim, o valor da nossa integral é:
Resposta
.
Exercícios de Livros Relacionados
Calcule ∫ x 2 1 - x 2 d x
Calcule ∫ - x 2 + 2 x + 2 d x
Calcule a área do conjunto de todos ( x , y ) tais que x ≥ 1 + y 2 e 2 x + y ≤ 2 .
Calcule a área do conjunto de todos ( x , y ) tais que x^2 + 2 y^2 = 3 e y = x^2 .
Os exercícios de 43 a 45 referem-se à Seção Suplementar 6.7.43. Uma tubulação horizontal cilíndrica tem um diâmetro interno de 4m e uma extremidade fechada por um registro circular que se ajusta à tub