Responde Aí
Acesse com GoogleAcesse com AppleAcesse com Facebook

Enunciado

Cálculo - Cálculo com Geometria Analítica Volume 1 - 3ª Edição - Louis Leithold- Ed: 3º - Capítulo 9.4 - Ex. 12

Calcule a integral indefinida:

int (d x)/(x^4 sqrt(16 + x^2))

Passo 1

Pessoal, a primeira coisa que devemos fazer aqui para resolver essa integral indefinida é saber qual substituição trigonométrica usar, como nós temos x^2 + 16 = x^2 + 4^2 no denominador, isso indica a substituição com a tangente, como vocês podem ver a seguir no quadro de substituições com os respectivos triângulos e intervalos.

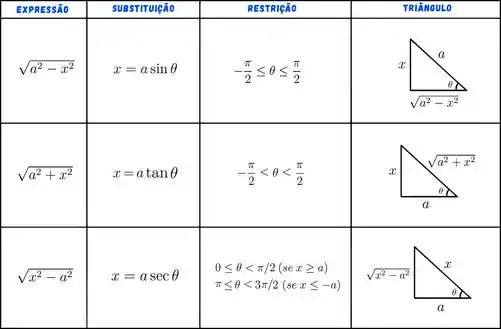
Olhando o quadrinho, percebemos que a substituição deve ser x = 4 tan theta .

Passo 2

Façamos então a substituição:

[[x,=,4,tan theta],[d,x,=,4,sec^2 theta,d,theta]]

Dessa forma, x^2 = 16 tan^2 theta -> x^2 + 16 = 16 tan^2 theta + 16 = 16 overset(sec^2 theta)(obrace (tan^2 theta + 1)) = 16 sec^2 theta .

Passo 3

Agora vamos trocar a variável da integral utilizando:

int (d x)/(x^4 sqrt(16 + x^2)) = int (4 sec^2 theta d theta)/(( 4 tan theta )^4 sqrt(16 sec^2 theta))

Considerando os intervalos adequados, podemos tirar o sec^2 theta da raiz sem o módulo.

int (4 sec^2 theta d theta)/(( 4 tan theta )^4 sqrt(16 sec^2 theta)) = 4 int (sec^2 theta d theta)/(4^4 tan^4 theta * 4 sec theta) = (4)/(4^5) int (sec^2 theta d theta)/(tan^4 theta sec theta) = (1)/(256) int (sec theta d theta)/(tan^4 theta)

= (1)/(256) int ((1)/(cos theta))/((sen^4 theta)/(cos^4 theta)) d theta = (1)/(256) int (cos^3 theta)/(sen^4 theta) d theta = (1)/(256) int (overset(cos^2 theta)(obrace (1 - sen^2 theta)) cos theta)/(sen^4 theta) d theta

Agora faremos uma substituição u = sen theta -> d u = cos theta d theta . Ficamos então com:

(1)/(256) int ((1 - sen^2 theta) cos theta)/(sen^4 theta) d theta = (1)/(256) int ((1 - u^2))/(u^4) d u = (1)/(256) int ((1)/(u^4) - (u^2)/(u^4)) d u

= (1)/(256) int ( u^(- 4) - u^(- 2) ) d u

Passo 4

Podemos resolver essa integral utilizando int u^n d u = (u^(n + 1))/(n + 1) + C n != - 1 .

(1)/(256) int ( u^(- 4) - u^(- 2) ) d u = (1)/(256) ((u^(- 4 + 1))/(- 4 + 1) - (u^(- 2 + 1))/(- 2 + 1)) + C = (1)/(256) ((u^(- 3))/(- 3) - (u^(- 1))/(- 1)) + C

= (1)/(256) (- (1)/(3 u^3) + (1)/(u)) + C

Passo 5

Como a integral indefinida foi dada na variável x , devemos dar a resposta nesta variável. Sendo x = 4 tan theta e sen theta = u , então:

int (d x)/(x^4 sqrt(16 + x^2)) = (1)/(256) (- (1)/(3 ( sen theta )^3) + (1)/(( sen theta ))) + C

Para achar sen theta nós olhamos o triângulo da substituição seno construído no inicio e utilizamos essas relações trigonométricas. Temos então:

sen theta = (x)/(sqrt(x^2 + a^2)) = (x)/(sqrt(x^2 + 16))

Dessa forma, o resultado da integral será:

int (d x)/(x^4 sqrt(16 + x^2)) = (1)/(256) (- (1)/(3 ((x)/(sqrt(x^2 + 16)))^3) + (1)/(((x)/(sqrt(x^2 + 16))))) + C

= (1)/(256) (- ((sqrt(x^2 + 16))^3)/(3 x^3) + (sqrt(x^2 + 16))/(x)) + C

= (1)/(256) (- (( x^2 + 16 ) sqrt(x^2 + 16))/(3 x^3) + (3 x^2 sqrt(x^2 + 16))/(3 x^3)) + C

= (1)/(256) ((- (x^2 + 16) sqrt(x^2 + 16) + 3 x^2 sqrt(x^2 + 16))/(3 x^3)) + C

= (1)/(768) (((3 x^2 - x^2 - 16) sqrt(x^2 + 16))/(x^3)) + C

= (1)/(768) (((2 x^2 - 16) sqrt(x^2 + 16))/(x^3)) + C = (2)/(768) (((x^2 - 8) sqrt(x^2 + 16))/(x^3)) + C

int (d x)/(x^4 sqrt(16 + x^2)) = ((x^2 - 8) sqrt(x^2 + 16))/(384 x^3) + C

Resposta

int (d x)/(x^4 sqrt(16 + x^2)) = ((x^2 - 8) sqrt(x^2 + 16))/(384 x^3) + C

Ver Outros Exercícios desse livro

Exercícios de Livros Relacionados

Calcule a integral. ∫ 4 - x 2 d x
Calcule a integral. ∫ 1 x 2 9 - x 2 d x
Calcule a integral. ∫ 1 - 4 x 2 d x
Calcule a integral. ∫ 1 + t 2 t d t
Calcule a integral. ∫ x 2 16 - x 2 d x