Responde Aí
Acesse com GoogleAcesse com AppleAcesse com Facebook

Enunciado

Cálculo - Cálculo com Geometria Analítica Volume 1 - 3ª Edição - Louis Leithold- Ed: 3º - Capítulo 9.4 - Ex. 7

Calcule a integral indefinida.

int (d x)/(sqrt(x^2 - a^2))

Passo 1

Primeiramente vamos pegar o sqrt(x^2 - a^2) e desenvolver a Identidade Pitagórica.

t = sqrt(x^2 - a^2)

t^2 = x^2 - a^2

t^2 + a^2 = x^2

Montando o triângulo temos

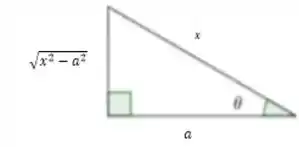
Com base nesse triângulo vamos usar a seguinte relação trigonométrica sec theta = (x)/(a)

Passo 2

Agora vamos aplicar a seguinte substituição chamar x = a sec theta e também as mudanças necessárias.

x = a sec theta

d x = a tan theta sec theta d theta

int (a tan theta sec theta)/((sqrt((a sec theta)^2 - a^2))) d theta

Passo 3

Vamos aplicar a Identidade Trigonométrica.

int (a tan theta sec theta)/((sqrt(( sec^2 theta - 1 ) a^2))) d theta

(cos^2 theta + sin^2 theta = 1) * (1)/(cos^2 theta)

1 + tan^2 theta = sec^2 theta

int (a tan theta sec theta)/(a tan theta) d theta

int sec theta d theta

Passo 4

Agora podemos resolver a integral e em seguida desfazer as mudanças através das relações trigonométricas do triângulo montado anteriormente sec theta = (x)/(a) e tan theta = (sqrt(x^2 - a^2))/(a) .

int sec theta d theta

ln (|(x)/(a) + (sqrt(x^2 - a^2))/(a)|) + c

ln (|(x + sqrt(x^2 - a^2))/(a)|) + c

ln (|x + sqrt(x^2 - a^2)|) - ln (|a|) + c

Resposta

ln (|x + sqrt(x^2 - a^2)|) - ln (|a|) + c

Ver Outros Exercícios desse livro

Exercícios de Livros Relacionados

Calcule a integral ∫ 0 1 x 2 + 1d x
Calcule a integralint_0^(0,6) (x^2)/(sqrt(9 - 25 x^2)) d x
Calcule a integral: ∫ 0 1 x x 2 + 4d x
Calcule a integral ∫ x 2 - a 2 x 4 d x
Calcule a integralint (d x)/([(a x)^2 - b^2]^((3)/(2)))