Responde Aí
Acesse com GoogleAcesse com AppleAcesse com Facebook

Enunciado

Cálculo - Cálculo Volume 1 - 7ª Edição - James Stewart- Ed: 7º - Capítulo 7.3 - Ex. 12

Calcule a integral

int (d u)/(u sqrt(5 - u^2))

Passo 1

Olá, meu nobre e querido amigo. Yuri aqui e estarei te ajudando a resolver essa atividade.

Veja bem, queremos aqui resolver essa integral indefinida. Vamos começar então pelo método mais elementar, e caso o mesmo não se mostre possível a gente vai pensando em outros métodos.

Nessa aqui, uma substituição simples não funciona, então irei lhe apresentar a substituição trigonométrica. O próprio livro tem uma tabela que ajuda bastante.

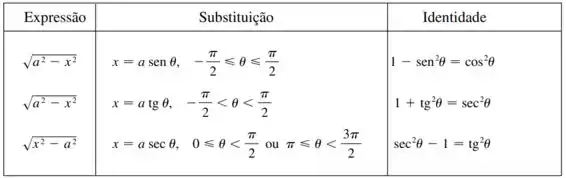
Passo 2

Para resolver essa integral iremos utilizar a seguinte forma sqrt(a^2 - x^2) . Então iremos substituir assim

u = sqrt(5) sin theta

d u = sqrt(5) cos theta d theta

Agora substituindo na integral:

int (d u)/(u sqrt(5 - u^2)) = int (sqrt(5) cos theta d theta)/(sqrt(5) sin theta sqrt(5 - (sqrt(5) sin theta)^2))

= int (cos theta d theta)/(sin theta sqrt(5 (1 - sin^2 theta)))

= (1)/(sqrt(5)) int (cos theta d theta)/(sin theta sqrt(cos^2 theta))

= (1)/(sqrt(5)) int (cos theta d theta)/(sin theta cos theta)

= (1)/(sqrt(5)) int (d theta)/(sin theta)

= (1)/(sqrt(5)) int csc theta d theta

Passo 3

Para resolver esta integral, vamos utilizar a identidade trigonométrica ( csc^2 x = cot^2 x + 1 ) . Agora, a integral dada se transforma em:

int (d u)/(u sqrt(5 - u^2)) = (1)/(sqrt(5)) int csc x d x

= (1)/(sqrt(5)) int sqrt(cot^2 x + 1) d x

= (1)/(sqrt(5)) int sqrt(cot^2 x + 1) ((- csc^2 x)/(- csc^2 x)) d x

= (- 1)/(sqrt(5)) int (sqrt(cot^2 x + 1))/(- csc^2 x) (- csc^2 x) d x

= - (1)/(sqrt(5)) int (sqrt(cot^2 x + 1))/(cot^2 x + 1) (- csc^2 x) d x

= - (1)/(sqrt(5)) int (sqrt(t^2 + 1))/(t^2 + 1) d t

= - (1)/(sqrt(5)) int (d t)/(sqrt(t^2 + 1))

Temos que a integral acima é tabelada

int (d u)/(u sqrt(5 - u^2)) = - (1)/(sqrt(5)) int (d t)/(sqrt(t^2 + 1))

= - (1)/(sqrt(5)) ln |t + sqrt(t^2 + 1)| + C

= - (1)/(sqrt(5)) ln | c t g theta + sqrt(c t g^2 theta + 1) | + C

Passo 4

Para obter o resultado final em termos de u , precisamos substituir theta por u . A partir do passo 2, temos:

u = sqrt(5) sin theta

(u)/(sqrt(5)) = sin theta

arcsin (u)/(sqrt(5)) = theta

Substituindo ( cot theta ) por ( cos theta // sin theta ) (também substituiremos ( cos^2 theta ) por ( sin^2 theta ) devido a ( a r c s i n ) ) , e em seguida, usando a substituição, obtemos o resultado final da seguinte forma:

int (d u)/(u sqrt(5 - u^2)) = - (1)/(sqrt(5)) ln | c t g theta + sqrt(c t g^2 theta + 1) | + C

= - (1)/(sqrt(5)) ln | (cos theta)/(sin theta) + sqrt((cos^2 theta)/(sin^2 theta) + 1) | + C

= - (1)/(sqrt(5)) ln |(sqrt(1 - sin^2 theta))/(sin theta) + sqrt((1 - sin^2 theta)/(sin^2 theta) + 1)| + C

= - (1)/(sqrt(5)) ln |(sqrt(1 - sin^2 theta))/(sin theta) + (1)/(sin theta)|

= - (1)/(sqrt(5)) ln |(1 + sqrt(1 - sin^2 theta))/(sin theta)|

= - (1)/(sqrt(5)) ln |(1 + sqrt(1 - sin^2 (arcsin ((u)/(sqrt(5))))))/(sin (arcsin ((u)/(sqrt(5)))))|

= - (1)/(sqrt(5)) ln |(1 + sqrt(1 - ((u)/(sqrt(5)))^2))/((u)/(sqrt(5)))|

= - (1)/(sqrt(5)) ln |(1 + sqrt((5 - u^2)/(5)))/((u)/(sqrt(5)))|

= - (1)/(sqrt(5)) ln |((sqrt(5) + sqrt(5 - u^2))/(sqrt(5)))/((u)/(sqrt(5)))|

= - (1)/(sqrt(5)) ln |(sqrt(5) + sqrt(5 - u^2))/(u)|

Resposta

= - (1)/(sqrt(5)) ln |(sqrt(5) + sqrt(5 - u^2))/(u)|

Ver Outros Exercícios desse livro

Exercícios de Livros Relacionados

Calcule a integral.int (1)/(x^2 sqrt(x^2 - 16)) d x
Calcule a integral.int (3 x^3)/(sqrt(1 - x^2)) d x
Calcule a integral.int (1)/(x^2 sqrt(9 x^2 - 4)) d x
Calcule a integral.int (x^2)/(sqrt(5 + x^2)) d x
Calcule a integral.int (1)/((4 + x^2)^2) d x