Enunciado
Cálculo - Diva Flemming - Cálculo B - Ed: 2º - Capítulo 7.8 - Ex. 5d
Usando coordenadas polares, calcular:
d)
Passo 1
Temos que:
A representação da região de integração é dada:
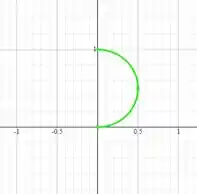
Assim:
Como:
; ;
Temos:
Desse modo, temos que:
Passo 2
As coordenadas polares se dão da seguinte forma:
; ;
Então, substituindo na equação dada, temos:
Passo 3
Integrando para :
Aplicando os limites:
Passo 4
Integrando para :
Aplicando os limites:
Resposta
Exercícios de Livros Relacionados
Calcular ∬_R^() sqrt((x - 1)^2 + (y - 2)^2) d x d y , onde R é delimitada por (x - 1)^2 + (y - 2)^2 = 1 .
Calcular ∬_R^() x y d x d y , onde R é delimitada por (x^2)/(4) + (y^2)/(9) = 1 .
Calcular ∬_R^() ln ( x^2 + y^2 ) d x d y , sendo R o anel delimitado por x^2 + y^2 = 16 e x^2 + y^2 = 25 .
Calcular ∬ R y d x d y , sendo R o círculo x 2 + y 2 - 4 y ≤ 0 .
Calcular ∬_R^() ( 8 - x - y ) d x d y , sendo R delimitada por x^2 + y^2 = 1 .