Responde Aí
Acesse com GoogleAcesse com AppleAcesse com Facebook

Enunciado

Mecânica e Resistência dos Materiais - Mecânica para Engenharia Estática - 12ª Edição - Russel C. Hibbeler- Ed: 12º - Capítulo 9.Problemas - Ex. 31

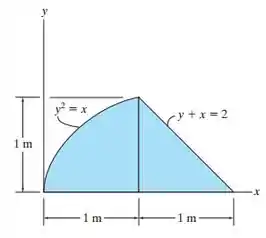
Localize o centroide da área. Dica: Escolha elementos de espessura d y e comprimento [ 2 - y - y 2 ] .

Passo 1

O exercício nos dá a dica de usar o elemento d y para termos uma região do Tipo II e não precisarmos dividir a região em duas. Vamos então tomar nosso elemento diferencial como a área dada por:

d A = ( x 2 - x 1 ) ⅆ y

Colocando nossa função em relação a y temos x 1 = y 2 , e x 2 = y - 2   , nosso d A fica:

d A = y - 2 - y 2   d x

Que é justamente a espessura sugerida pelo exercício.

Para o centroide do nosso elemento diferencial, em x - temos:

x - = x 2 + x 1 2 = 2 - y + y 2 2

E para y - temos o próprio y - = y .

Passo 2

Vamos encontrar nossa área:

A = ∫ A ⅆ A

A = ∫ 0 1 y - 2 - y 2   ⅆ y = 2 y - y 2 2 - y 3 3 0 1 = 1,167   m 2

Passo 3

E agora encontramos nosso centroide x - :

x - = ∫ A x - ⅆ A ∫ A ⅆ A

Temos:

∫ A x - ⅆ A = ∫ 0 1 2 - y + y 2 2 y - 2 - y 2   ⅆ y = 1 2 4 y - 2 y 2 + y 3 3 - y 5 5 0 1 = 1,067   m 3

Logo, nosso x - será:

x - = 1,067 1,167   ∴ x - = 0,914   m

E para nosso centroide y - :

y - = ∫ A y - ⅆ A ∫ A ⅆ A

Tendo:

∫ A y - ⅆ A = ∫ 0 1 y y - 2 - y 2   ⅆ y = y 2 - y 3 3 - y 4 4 0 1 = 0,4167   m 3

Assim, nosso y - será:

y - = 0,4167 1,167 ∴ y - = 0,357   m

Resposta

x - = 0,914   m

y - = 0,357   m

Ver Outros Exercícios desse livro

Exercícios de Livros Relacionados

5.26. Determine the y-coordinate of the centroid of the shaded area shown.
5.30. Determine the z-coordinate of the centroid of the volume generated by revolving the shaded area around the z-axis through 360°.
5.31. The figure represents a flat piece of sheet metal symmetrical about axis A-A and having a parabolic upper boundary. Choose your own coordinates and calculate the distance from the base to the ce
5.25. Use the results of Sample Problem 5/3 to compute the coordinates of the mass center of the portion of the solid homogeneous cylinder shown.
5.27. If the shaded area of Prob. 5/26 is rotated about the y-axis, determine the y-coordinate of the centroid of the resulting volume.