Responde Aí
Acesse com GoogleAcesse com AppleAcesse com Facebook

Enunciado

Cálculo - Um curso de Cálculo Volume 2 - 5ª Edição - Hamilton Luiz Guidorizzi- Ed: 5º - Capítulo 3.6 - Ex. 1b

Determine o centro de massa da região A dada

b ) A = {(x , y) in R | x^2 + 4 y^2 <= 1 , x >= 0 , y >= 0}

Passo 1

Para encotrarmos o centro de massa precisamos achar x_c e y_c que são descritos por:

x_c = (int_a^b x [g (x) - f (x)] d x)/(Á r e a d e A)

y_c = (1)/(2) (int_a^b [g (x) + f (x)] [g (x) - f (x)] d x)/(Á r e a d e A)

A figura fica assim:

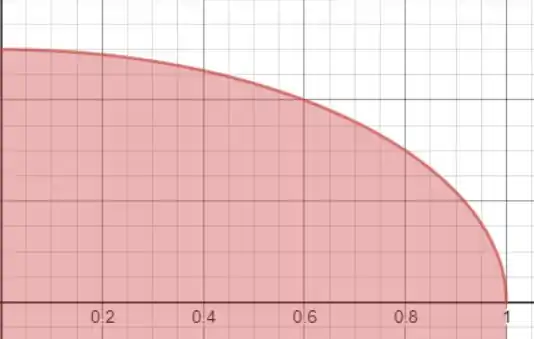
Daí vamos achar uma função y em função de x :

y (x) = (1)/(2) sqrt(1 - x^2)

Agora vamos achar a Área de A:

int_0^1 (1)/(2) sqrt(1 - x^2) d x

Vamos agora calcular.

Passo 2

(1)/(2) int_0^1 sqrt(1 - x^2) d x

Vamos usar substituição trigonométrica para esse caso. Imagine que temos o triângulo abaixo:

E seu cateto adjacente a theta vale sqrt(1 - x^2) e o cateto oposto vale x com a hipotenusa valendo 1. Assim as seguintes relações valem:

cos theta = sqrt(1 - x^2) e sin theta = x -> d x = cos theta d theta

Fazendo as substituições:

(1)/(2) int_0^((pi)/(2)) cos^2 theta d theta = (1)/(2) int_0^((pi)/(2)) (1)/(2) (1 + cos 2 theta) d theta = (1)/(4) [theta + (sin 2 theta)/(2)] ((pi)/(2))/(0) = ((pi)/(8))

Agora que temos a Área de A vamos achar as outras integrais:

int_a^b x [g (x) - f (x)] d x = (1)/(2) int_0^1 x sqrt(1 - x^2) d x =

Vamos fazer aqui a substituição: u = 1 - x^2 -> d u = - 2 x d x

(1)/(2) int_0^1 x sqrt(1 - x^2) d x = - (1)/(4) int_1^0 sqrt(u) d u = - (1)/(4) [(2)/(3 sqrt(u))] (0)/(1) = - (1)/(4) [- (2)/(3)] = (1)/(6)

int_a^b [g (x) + f (x)] [g (x) - f (x)] d x = (1)/(4) int_0^1 (sqrt(1 - x^2)) (sqrt(1 - x^2)) d x

= (1)/(4) int_0^1 (1 - x^2) d x = (1)/(4) [x - (x^3)/(3)] (1)/(0) = (1)/(4) (1 - (1)/(3) (1)^3) = (1)/(4) (1 - (1)/(3)) = ((1)/(4) xx (2)/(3)) = ((1)/(6))

Passo 3

Agora substituímos:

x_c = (int_a^b x [g (x) - f (x)] d x)/(Á r e a d e A) = ((1)/(6))/((pi)/(8)) = (4)/(3 pi)

y_c = (1)/(2) (int_a^b [g (x) + f (x)] [g (x) - f (x)] d x)/(Á r e a d e A) = (1)/(2) ((1)/(6))/((pi)/(8)) = (1)/(2) xx (2)/(3 pi) = (2)/(3 pi)

Resposta

(x_c , y_c) = ((4)/(3 pi) , (2)/(3 pi))

Resposta

Ei, a resposta está no passo a passo :)

Ver Outros Exercícios desse livro

Exercícios de Livros Relacionados

Calculando as integrais I 1 = ∫ 1 2 x 2 d x , I 2 = ∫ 1 2 x d x e I 3 = ∫ 1 2 d x , obtemos I 1 = 7 3 , I 2 = 3 2 e I 3 = 1 . Usando esses resultados, encontre o valor de:d) ∫ 1 2 3 x + 2 2 d x
24. Obtenha ∫ - 2 3 f x d x se ∫ - 2 1 f x d x = - 2 e ∫ 3 1 f x d x = 1
21. Obtenha ∫ - 1 2 f x + 2 g ( x ) d x se ∫ - 1 2 f x d x = 5 e ∫ - 1 2 f x d x = - 3 .
23. Obtenha ∫ 1 5 f x d x se ∫ 0 1 f x d x = - 2 e ∫ 0 5 f x d x = 1
25-28. Use o Teorema 5.5 . 4 e fórmulas apropriadas de Geometria para calcular as integrais. 26 . ∫ - 2 2 1 - 3 x d x