Enunciado
Cálculo - Um curso de Cálculo Volume 1 - 5ª Edição - Hamilton Luiz Guidorizzi- Ed: 5º - Capítulo 7.15 - Ex. 7
Seja .
a) Estude o sinal de .
b) Estude o sinal de .
c) Calcule e .
d) Utilizando as informações acima, esboce o gráfico de .
Passo 1
a) Derivando temos:
Temos que a expressão descreve uma parábola cuja concavidade está voltada para cima, assim, sua parte negativa está entre as raízes e a parte positiva está na região externa às raízes.
Como as raízes são:
Ou
Então, para , para e para ou .
Passo 2
b) Derivando temos:
Como é uma reta crescente, a parte positiva está depois da sua raiz e a negativa está antes.
Como sua raiz é:
Então, para , para e para .
Passo 3
c) Para calcular e , devemos por em evidência:
Assim:
E
Logo, e .
Passo 4
Com as informações coletadas, temos então o seguinte gráfico:
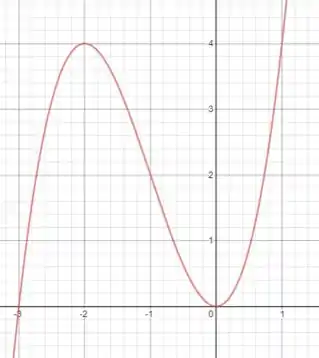
Resposta
a) para , para e para ou .
b) para , para e para .
c) e .
Exercícios de Livros Relacionados
Um objeto move-se ao longo de uma reta, de acordo com a equação de movimento s = 5 + t 2 , com t ≥ 0 . Ache o valor de t para o qual a medida da velocidade instantânea é (a) 0 ; (b) 1 .
Um objeto move-se ao longo de uma reta, de acordo com a equação de movimento s = 4 t 2 + 3 , com t ≥ 0 . Ache o valor de t para o qual a medida da velocidade instantânea é (a) 0 ; (b) 1 ; (c) 2 .
Suponha que um líquido seja produzido por um certo processo químico e que a função custo total C seja dada por C x = 6 + 4 x , onde C ( x ) é a quantia correspondente ao custo total da produção de x l
A quantia em dinheiro no custo total da produção de x unidades de certa mercadoria é dada por C x = 40 + 3 x + 9 2 x . Ache (a) o custo marginal quando 50 unidades são produzidas e (b) o número de uni
Uma imobiliária que administra um condomínio aluga cada apartamento por $ p por mês quando x apartamentos são alugados por p = 30 300 - 2 x . Se $ R ( x ) for o rendimento recebido do aluguel de x apa